Since P(x) = R(x) - C(x), then:
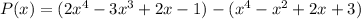
Then, simplify the expression above.
First, distribute the negative sign to the terms inside the second parenthesis.
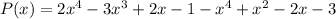
Then, arrange the terms according to their degree.
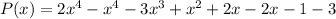
Then, combine similar terms.
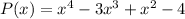
Therefore, the value P(x) is x⁴ - 3x³ + x² - 4.