Answer:
The amount of money that will be in the account after 8 years is $1513.13
Explanations:
Amount Invested is the Principal, P
P = $1500
Interest Rate, r = 3.5% = 3.5/100 = 0.035
The interest was compounded quarterly
There are 4 quarters in a year
That is, n = 44
The total number of years, t = 8
The amount formula for compound interest is:
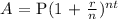
To get the amount of money that will be in the account after 8 years, substitute r = 0.035, n = 4, and P = $1500 into the formula above:

A = 1500( 1 + 0.00875)
A = 1500( 1.00875)
A = 1513. 13
Therefore, the amount of money that will be in the account after 8 years is $1513.13