Question

Find an equation of variation in which y varies inversely as x and y=50 and x=42. Then find the value of y when x=30.

Answer 1

When two variables are inversely proportional as one of them increase the other will decrease. This means that there is a constant number that relates these two variables and that is less than 1. This can be expressed in a general form with the following expression:

`y\cdot x=k`

We need to determine the value "k", which is the constant of proportionality, it is the number that determines how much the variations on one variable will affect the other. We can determine this constant by replacing "y" and "x" by known numbers, which for our case are 50 and 42. We do this below:

`\begin{gathered} 50\cdot42=k \\ k=2100 \end{gathered}`

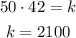
With the value of k we can write the correct expression:

`x\cdot y=2100`

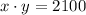
If we replace the value of x by 30, we can find the value of y.

`\begin{gathered} 30\cdot y=2100 \\ y=(2100)/(30)=70 \end{gathered}`

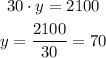
The value of y when x=30 is 70.

The equation is:

`y=(2100)/(x)`