recall that a polynomial can be written as the multiplication of multiple factors, namely of the form

where c is a zero of the function. Note that when having a complex zero, we must have the complex conjugate as another zero. Recall that given a complex number of the form a+bi, the complex conjugate is a-bi. Recall that
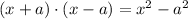
So will calculate the product of each pair of zeros:
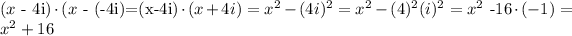
in the same manner, we would have
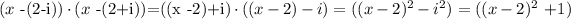
so we have that our polynomial would be
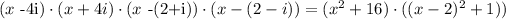
Now we only need to calculate the product of the right. So we have
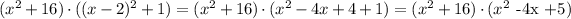
now we distribute to get

which would be our final answer