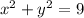Question

Read the equation in standard form for the circle passing through (-3,0) centered at the origin.

Answer 1

the standard equation of circles is:

`(x-h)^2+(y-k)^2=r^2`

in which the circle is centered at (0,0).

Since the circle is centered at the origin (h,k) is (0,0), then, the equation can be reduced to:

`x^2+y^2=r^2`

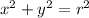
then, since the circle passes through the point (-3,0) the radius is equal to 3,

finally, the standard equation can be replaced with the radius

`\begin{gathered} x^2+y^2=3^2 \\ x^2+y^2=9 \end{gathered}`

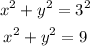
Answer:

The equation for a circle centered at the origin that passes through the point (-3,0) is:

`x^2+y^2=9`