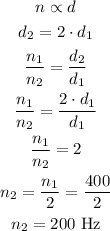ANSWER:
(a) 355.56 Hz
(b) 282.84 Hz
(c) 309.84 Hz
(d) 200 Hz
Explanation:
Given:
n1 = primary frequency = 400 Hz
L1 = primary length = 0.8 m
(a)
New length = L2 = 0.9 m
Frequency of the length is string isproportional to the length of the string. i.e n ∝ L1
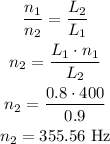
(b)
ρ is the density
![\begin{gathered} n\propto(1)/(√(\rho)) \\ \rho_2=2\rho_1 \\ (n_1)/(n_2)=\sqrt{(\rho_2)/(\rho_1)} \\ (n_1)/(n_2)=\sqrt[]{(2\rho_1)/(\rho_1)} \\ (n_1)/(n_2)=\sqrt[]{2} \\ n_2=\frac{n_1}{\sqrt[]{2}}=\frac{400}{\sqrt[]{2}} \\ n_2=282.84\text{ Hz} \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/d4jckj3k3n1bc5f2kyunb3ga87lt4swugj.png)
(c)
T is the tension
![\begin{gathered} n\propto\sqrt[]{T} \\ T_2=T_1-0.4\cdot T_1 \\ T_2=0.6\cdot T_1 \\ (n_2)/(n_1)=\sqrt[]{\frac{T_2}{T_1_{}}} \\ (n_2)/(n_1)=\sqrt[]{(0.6\cdot T_1)/(T_1)} \\ (n_2)/(n_1)=\sqrt[]{0.6} \\ n_2=400\cdot\sqrt[]{0.6} \\ n_2=309.84\text{ Hz} \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/1bztvt9am6fj9x415zj816dnfn0w8uywvh.png)
(d)
d is the diameter