Question

Point L is located in AB such that the ration of AP:PB is 1:2. If the coordinates of the AB are A(-2, 4) and B(7, -2), what is the x-coordinate of point P?

Answer 1

Given: The line segment has the co-ordinates are A (-2,4) and B( 7,-2).

To find: The x -coordinate of the point P which divides the line segment in the ratio 1:2.

Explanantion: Suppose we have line segment AB and a point divided it into ration a:b then the general form for determining the coordinates of the point P are :

`\langle((a)/(a+b)(x_2-x_1)),(a)/(a+b)(y_2-y_1)\rangle`

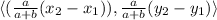
The final coordinate is

`\begin{gathered} x=x_1+(a)/(a+b)(x_2-x_1) \\ =(bx_1+ax_2)/(a+b) \\ y=y_1+(a)/(a+b)(y_2-y_1)_ \\ =(by_1+a_(y2))/(a+b) \end{gathered}`

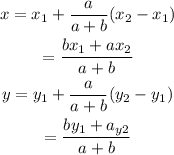
Here , in the provided case (x1,y1) and (x2,y2) are (-2,4) and (7,-2).

and the ration is a:b=1:2.

so, we get a=1 and b=2.

Plug the values of the coordinates and the a and b in the above stated formula to get:

`\begin{gathered} x=(2*-2+1*7)/(1+2) \\ =(-4+7)/(3) \\ =(3)/(3) \\ =1 \end{gathered}`

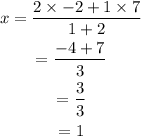
The value of x-coordinate of point P is x=1.

Final Answer: The x-coordinate is x=1.