Question

For this you're supposed to sort them and see if they are a (solution) or a (none solution) But I'm a little confused how to do this.So I just need help with this.

Answer 1

The given inequality is

`y>3x-2`

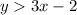
We have to evaluate each point in order to determine which one is a solution, and which one is not. Remember that the first number of a pair represents the value for x, and the second number represents the value for y.

(-7, 4).

`\begin{gathered} 4>3(-7)-2 \\ 4>-21-2 \\ 4>-23 \end{gathered}`

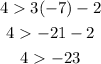
Notice that the result is true, 4 is more than -23. Hence, (-7, 4) is a solution.

(1, 0).

`\begin{gathered} 0>3(1)-2 \\ 0>3-2 \\ 0>1 \end{gathered}`

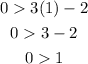
Zero is not more than 1, this result is false, which means (1,0) is not a solution.

(1, 6).

`\begin{gathered} 6>3(1)-2 \\ 6>3-2 \\ 6>1 \end{gathered}`

This result is true because 6 is greater than 1. Therefore, (1,6) is a solution.

(0, -2).

`\begin{gathered} -2>3(0)-2 \\ -2>-2 \end{gathered}`

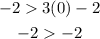
-2 is not greater than -2, they are equal, so (0, -2) is not a solution.

(4, -4).

`\begin{gathered} -4>3(4)-2 \\ -4>12-2 \\ -4>10 \end{gathered}`

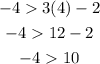
-4 is not greater than 10, this result is false. Therefore, (4, -4) is not a solution.

In sum, the solutions are (-7, 4) and (1, 6).