Let l be the length of the rectangle and w be the width, since the length is five inches more than the width we can set the following equation:
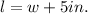
Also, the perimeter of the rectangle is 50 inches, therefore:
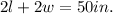
Substituting the first equation in the second equation we get:
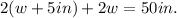
Solving for w we get:
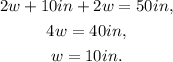
Substituting w=10in in the first equation we get:
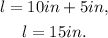
Answer: The length of the rectangle is 15 inches and the width is 10 inches.