Question

We wish to estimate what percent of adult residents in a certain county are parents. Out of 200adult residents sampled, 176 had kids. Based on this, construct a 99% confidence interval for theproportion, p, of adult residents who are parents in this county.Need lower bound and upper bound

Answer 1

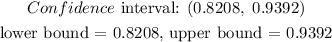
`\begin{gathered} Confidence\text{ interval: (}0.8208,\text{ 0.9392}) \\ \text{lower bound = 0.8208, upper bound = 0.9392} \end{gathered}`Step-by-step explanation:
`\begin{gathered} \text{Total adult residents = 200} \\ \text{Number that had kids = 176} \\ Point\text{ estimate = }(176)/(200)\text{ = }0.88 \\ Point\text{ estimate = sample proportion (p) = 0.88} \end{gathered}`

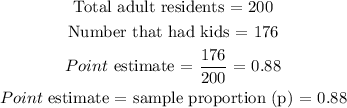
From a standard normal table, the z score for a 99% confidence interval = 2.576

Calculating margin of error:

`\begin{gathered} E=Z_{score\text{ }}*\text{ }\sqrt[]{(p(1-p))/(n)} \\ E\text{ = margin of error} \\ Z_{score\text{ }}=\text{ 2.576},\text{ p = 0.88},\text{ n = 200} \\ E=2.576*\text{ }\sqrt[]{(0.88(1-0.88))/(200)} \\ E\text{ = }2.576*\text{ }\sqrt[]{(0.88(0.12))/(200)}\text{ = }2.576*\text{0}.02298 \\ E\text{ = 0.0592} \end{gathered}`

[tex]\begin{gathered} Confidence\text{ interval: (p - E, p + E)} \\ Confidence\text{ interval: (0.88 - 0.0592, 0.88 + 0.0592)} \\ Confidence\text{ interval: (}0.8208,\text{ 0.9392}) \\ \\ \text{Confidence interval in tri-inequality form: 0.88 - 0.0592 < p < 0.88 + 0.0592} \\ 0.8208