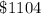Question

The polynomial C ( x ) = 6 x^2 + 90x gives the cost, in dollars, of producing a rectangular container whose top and bottom are squares with side x feet and height 6 feet. Find the cost of producing a box with x = 8 feet. The cost is $

Answer 1

Given the equation:

`C(x)=6x^2+90x`

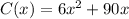
You know that "C" is the cost (in dollars) of producing a rectangular container, and "x" is the length of the side of the top and bottom that are squares.

In order to find the cost (in dollars) of producing a box container with a side of 8 feet, you need to set up that:

`x=8`

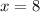
Then, you have to substitute that value of "x" into the equation and then evaluate:

`\begin{gathered} C(8)=6(8)^2+90(8) \\ \\ C(8)=6(64)+720 \end{gathered}`
`\begin{gathered} C(8)=6(64)+720 \\ \\ C(8)=384+720 \end{gathered}`
`C(8)=1104`

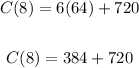
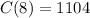
Hence, the answer is:

`\text{ \$}1104`