We have the following equation
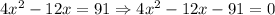
We can solve it using the quadratic formula:
![x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/rxvf73usjbbwyik14knxdemoz21vfz2ufc.png)
Where, in our problem
a = 4
b = -12
c = -91
Therefore
![\begin{gathered} x=\frac{-(-12)\pm\sqrt[]{(-12)^2-4\cdot4\cdot(-91)}}{2\cdot4} \\ \\ x=\frac{12\pm\sqrt[]{144+16\cdot91)}}{8} \\ \\ x=\frac{12\pm\sqrt[]{144+1456}}{8} \\ \\ x=\frac{12\pm\sqrt[]{1600}}{8} \\ \\ x=(12\pm40)/(8) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/l8ugsn8v76o00yppkl8eonb3cgn3icqop1.png)
Now we have the solutions, we only want the negative one, then
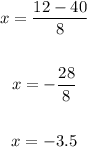
The negative solution of the equation is -3.5, then, the correct answer is the letter A.