To answer this question, we need to rewrite the equations into the slope-intercept form of the line:

Then, we have the first equation:
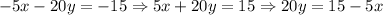
Then, we have:

For the second equation, we have:
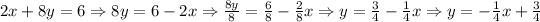
As we can see, both lines have the same equation. It means that if we graph both lines, the graph of both equations will be the same.
Let us find the x-intercept and the y-intercept of the lines (they are the same for both cases.)
The x-intercept
The x-intercept is the point for the line when y = 0. Then, when y = 0, we have:
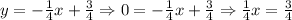
If we multiply by 4 to both sides of the equation, we have:
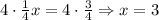
Then, the x-intercept is x = 3, y = 0 or (3, 0).
The y-intercept