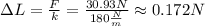In order to determine the elongation of the spring, proceed as follow:
Consider that the weight plus the elastic force on the block is equal to the buoyant force of the water:
W + F = E
where:
W: weight of the block = m*g = (5.71kg)(9.8m/s^2) = 55.96N
F: elastic force = kΔL
k: spring constant = 180N/m
E: buoyant force = m*g*ρw/ρb
Then, the net force on the block can be written as follow:
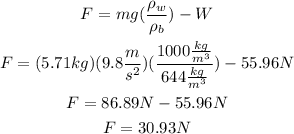
Next, use the previous value into the formula for the elastic force (Hooke's law) and solve for ΔL: