Question

Identify the y-intercept of the line containing M. Use the slope and they-intercept to write the equation of this line.

Answer 1

To be able to find the y-intercept and the equation of the line of JM, let's identify at least two points that the pass-through line JM and use it in getting the slope, y-intercept, and equation in Slope-Intercept Form: y = mx + b.

Let's use Point J and Point L.

Point J = x1,y1 = (0,6)

Point L = x2,y2 = (6,2)

Step 1: Let's determine the slope of the line (m).

`m\text{ = }\frac{y_2-y_1}{x_2-x_1_{}}`
`m\text{ = }\frac{2\text{ - 6}}{6\text{ - 0}}\text{ = }(-4)/(6)\text{ = -}((4)/(2))/((6)/(2))`
`m\text{ = -}(2)/(3)`

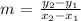
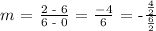
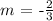
Step 2: Let's determine the y-intercept (b). Substitute m = -2/3 and x,y = 0,6 in y = mx + b.

`\text{ y = mx + b}`
`\text{ 6 = (-}(2)/(3))(0)\text{ + b}`
`\text{ 6 = 0 + b}`
`\text{ b = 6}`

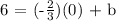
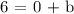
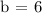
Step 3: Let's complete the equation. Substitute m = -2/3 and b = 6 in y = mx + b.

`\text{ y = mx + b}`
`\text{ y = (-}(2)/(3))x\text{ + (6)}`
`\text{ y = -}(2)/(3)x\text{ + 6}`

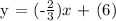
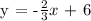
Therefore, the y-intercept of the line containing M is 6 and the equation of the line is y = -2x/3 + 6.