the distance from point A to point C is 21.26 km
Step-by-step explanation:
We need to find the distance from point A to point C. To get this, we need to know the angle facing AC
65° + ∠CBA = 180° (sum of angles on a line)
∠CBA = 180 - 65
∠CBA = 115°
To solve for the distance, let's make an illustration of the information we have:
Now we can use cosine rule to find the distance AC:
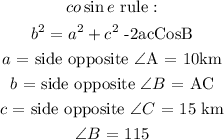
substitute the values into the formula:
![\begin{gathered} AC^2=10^2+15^2\text{ - 2(10)(15)}*\cos \text{ 115}\degree \\ AC^2\text{ = 100 + 225 - 300(-0.4226)} \\ AC^2\text{ = 325 + }126.78 \\ AC^2\text{ = 45}1.78 \\ AC\text{ = }\sqrt[]{\text{ 45}1.78} \\ AC\text{ = }21.2551 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/36vjw952w4xsxb8dhckh1tk2hsitirko9m.png)
To two decimal point, the distance from point A to point C is 21.26 km