To answer this question we will set and solve equations.
1) Notice that the angles that measure 2x+18 degrees and 3x-7 degrees are vertical angles. Since the lines with the marks are parallel, then:
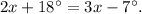
Adding 7degrees-2x to the above result we get:
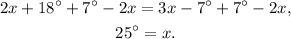
2) Notice that the angles that measure 78 degrees and y+10 degrees are corresponding angles. Since the lines with the marks are parallel, then:
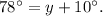
Subtracting 10 degrees from the above result we get:
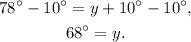
3) Recall that the interior angles of a triangle add up to 180 degrees, therefore:

Substituting the above result we get:
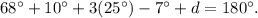
Simplifying the above result we get:
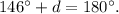
Therefore:
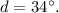
Answer:
