Question

Round each z-score to the nearest hundredth.A data set has a mean of x = 73 and a standard deviation of 10.8. Find the z-score for each of the following.(a) x = 85 _____(b) x = 95 _____(c) x = 50 _____(d) x = 75 _____

Answer 1

To determine the z-score of a raw data x, we have the formula below:

Based on the question, our mean is 73 and standard deviation is 10.8.

a. x = 85 (raw data is 85)

Let's plug these values to the z formula.

`z=(85-73)/(10.8)=(12)/(10.8)\approx1.11`

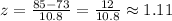
We subtracted the mean from the raw data first (85 - 73 = 12). Then, we divide the results by 10.8. The result is 1.11.

Hence, equivalent z-score of x = 85 is 1.11.