Statement Problem: Find the other legs of a 45-45-90 right triangle whose hypotenuse side is;
![10\sqrt[]{2}](https://img.qammunity.org/2023/formulas/mathematics/college/36jmhdbxv1tvlw766k6a9i9hex2ysbzj9u.png)
Solution:
Since the base angles are equal to 45 degrees, then the legs are equal. So, the opposite side is equal to the adjacent side.
Let the adjacent side and opposite side be x;
Now, we would apply the pythagoras theorem stated as;
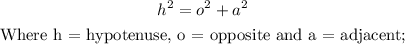
Thus, we have;
![\begin{gathered} (10\sqrt[]{2})^2=x^2+x^2 \\ 100(2)=2x^2 \\ 2x^2=200 \\ x^2=(200)/(2) \\ x^2=100 \\ x=\sqrt[]{100} \\ x=10 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/7n2oqbm0fbwov6nte2spk1qvoob86kw9oz.png)
CORRECT OPTION: C, none of these