Question

The last row please, calculate final velocities assuming perfectly Inelastic collision,

Answer 1

Given data:

* The mass of the first object is M_1 = 50 kg.

* The initial velocity of the first object is v_1i = 2 m/s.

* The mass of the second object is M_2 = 50 kg.

* The initial velocity of the second object is v_2i = 0 m/s.

Solution:

The initial momentum of the system is,

`\begin{gathered} p_i=M_1v_(1i)+M_2v_(2i) \\ p_i=50*2+50*0 \\ p_i=100+0 \\ p_i=100\text{ kgm/s} \end{gathered}`

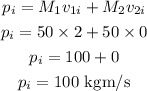
The final momentum of the system is,

`p_f=(M_1+M_2)v_{}`

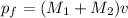
where v is the velocity of both the objects as both the objects are stick together after the collision(pure inelastic collision) and moves with the same velocity,

Substituting the known values,

`\begin{gathered} p_f=(50+50)v_{} \\ p_f=100v \end{gathered}`

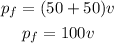
According to the law of conservation of momentum,

`\begin{gathered} p_i=p_f \\ 100=100v \\ v=(100)/(100) \\ v=1\text{ m/s} \end{gathered}`

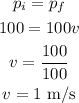
Thus, the final velocity of each object is 1 meter per second.