Question

Which equation represents the values in the table? x–1012y–13711A.y = 4x + 3B.y = −x − 1C.y = 3x − 1D.y = 1/4x − 34/

Answer 1

The equation is a linear equation

Let us pick any two points on the table.

When x = -1, y = -1 that is point (-1, -1)

When x = 0, y = 3, that is point(0, 3)

`\begin{gathered} \text{slope,m = }(y_2-y_1)/(x_2-x_1) \\ \text{where (}x_1,y_1)\text{ = (-1,-1)} \\ \text{and (x}_2,y_2)=(0,3) \end{gathered}`
`\begin{gathered} m=(3-(-1))/(0-(-1)) \\ m=(3+1)/(0+1) \\ \\ m=(4)/(1) \\ m=4 \end{gathered}`

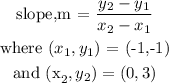
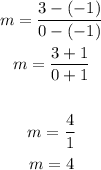
The eqaution od a straight line can be determined by the equation

`\begin{gathered} y-y_1=m(x-x_1) \\ y-(-1)=4(x-(-1) \\ y+1=4(x+1) \\ y+1=4x+4 \\ y=4x+4-1_{} \\ y=4x+3 \end{gathered}`

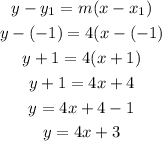
Hence, the answer is y= 4x +3. Option A is the answer