Parallel lines have equal slope.
Perpendicular lines have slopes that are negative reciprocals of each other.
Now, let us find the slope of each line.
Remember, the line's equation expression is :

Now,
Line 1:
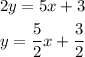
Slope if 5/2
Line 2:
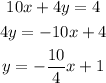
SLope is -10/4, or -5/2
Line 3:
y = 2/5x + 5
We don't have to rearrange, so the slope is 2/5
Now,
Answer choices
LIne 1 and Line 3 = neither (slope of 2/5 and 5/2 is neither same nor negative reciprocal)
Line 2 and Line 3 = Perpendicular. THey are exactly the NEGATIVE RECIPROCAL
Line 1 and Line 2 = they are same magnitude but different sign. Doesn't make sence.
SO, they are neighther.