Answer:
The frequency of the radiation = 10GHz
Step-by-step explanation:
The wavelength of the electromagnetic radiation, λ = 3.5 cm
λ = 3.5/100 m
λ = 0.035 m
The speed of light, c = 3 x 10⁸ m/s
Calculate the frequency (f) of the radiation using the formula:
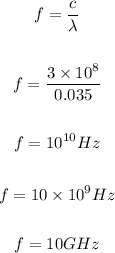
The frequency of the radiation = 10GHz