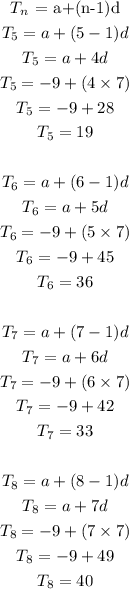Question

Please help I need this by the end of the day

Answer 1

no.9

this question deals with progression and it's a simple example of GP

to solve this question, let's find two things

1. first term

2. common difference

first term = 2

common ratio = 2

`\begin{gathered} T_7=ar^6 \\ T_7=2*2^6 \\ T_7=2*64 \\ T_7=128 \\ \\ T_8=ar^7 \\ T_8=2*2^7 \\ T_8=2*128 \\ T_8=256 \end{gathered}`

10. this question relates to arithmetic progression

`\begin{gathered} first\text{ term = 66} \\ \text{common difference = 16} \\ T_n=a+(n-1)d \\ T_5=66+(5-1)*16 \\ T_5=66+4*16 \\ T_5=66+64 \\ T_5=130 \\ \\ T_6=66+(6-1)*16 \\ T_6=66+5*16 \\ T_6=66+80 \\ T_6=146 \\ \\ T_7=66+(7-1)*16 \\ T_7=66+6*16 \\ T_7=66+96 \\ T_7=162 \\ \\ T_8=66+(8-1)*16 \\ T_8=66+7*16 \\ T_8=66+112 \\ T_8=178 \end{gathered}`

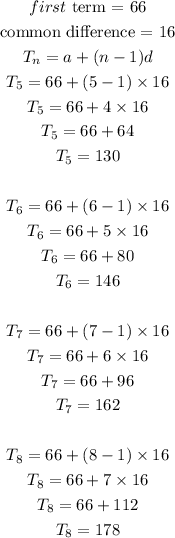
11. This question relates to geometric progression

first term(a) = 160

common ratio (r) = 1/2

`\begin{gathered} T_n=ar^(n-1) \\ T_5=160*((1)/(2))^(5-1) \\ T_5=160*(0.5)^4 \\ T_5=160*(1)/(16) \\ T_5=10 \\ \\ T_6=ar^(6-1) \\ T_6=160*((1)/(2))^5 \\ T_6=160*(1)/(32) \\ T_6=5 \\ \\ T_7=ar^(7-1) \\ T_7=ar^6 \\ T_7=160*((1)/(2))^6 \\ T_7=160*(1)/(64) \\ T_7=(5)/(2)=2.5 \\ \\ \\ T_8=ar^(8-1) \\ T_8=ar^7 \\ T_8=160*((1)/(2))^7 \\ T_8=160*(1)/(128) \\ T_8=\text{ }(5)/(4)=1.25 \end{gathered}`

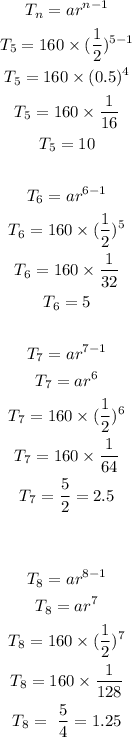
12. This question relates to arithmetic progression

first term (a) = -9

common difference(d) = 7

`\begin{gathered} T_n\text{ = a+(n-1)d} \\ T_5=a+(5-1)d \\ T_5=a+4d \\ T_5=-9+(4*7) \\ T_5=-9+28 \\ T_5=19 \\ \\ T_6=a+(6-1)d \\ T_6=a+5d \\ T_6=-9+(5*7) \\ T_6=-9+45 \\ T_6=36 \\ \\ T_7=a+(7-1)d \\ T_7=a+6d \\ T_7=-9+(6*7) \\ T_7=-9+42 \\ T_7=33 \\ \\ T_8=a+(8-1)d \\ T_8=a+7d \\ T_8=-9+(7*7) \\ T_8=-9+49 \\ T_8=40 \end{gathered}`