In order to calculate the surface area of the figure, we just have to find the area of each face and then, sum them up.
A triangular prism has 5 faces, 4 of them are rectangular faces, the area of a rectangle is given by the following formula:
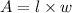
Where l is the length and w is the width.
The other two faces are triangular, we can calculate their area like this:
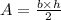
Where b is the base and h is the height of the triangle.
In this case, the faces on the sides of the prism (the diagonal faces) are squares whose length equals 11 in and their width equals 17, then the area of these faces is:
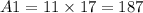
For the triangular faces, h equals 15 in and b equals 16 in, then the area of these faces is:
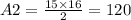
For the bottom face, whose dimensions are 11 and 16 in, we get:
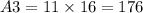
Now that we know the areas of each faces, we just have to sum them up, notice that since there are two triangular faces and two faces on the sides of the triangle we have to add twice A2 and A1, then we get:
A = A1 + A1 + A2 + A2 + A3
A = 2A1 + 2A2 + A3 = 2*187 + 2*120+ 176 = 790
Then, the surface area of the prism equals 790 square inches