Question

TThe Education Trust publishes data on U.S. colleges and universities. Six-year graduation rates and student-related expenditures per full-time student for 2007 were reported for the seven primarily undergraduate public universities in California with enrollments between 10,000 and 20,000. Suppose that a 90% confidence interval for the prediction of student expenditures at a university with a graduation rate of 40% was (5,304, 8,686).

Required:
a. Determine the point estimate for the prediction of student expenditures at a university with a graduation rate of 40%.
b. Calculate the margin of error.
c. Calculate the standard error of the prediction of student expenditures at a university with a graduation rate of 40%.

Answer 1

a) The point estimate is 6995.

b) The margin of error is of 1691.

c) The standard error is of 1000

Explanation:

a. Determine the point estimate for the prediction of student expenditures at a university with a graduation rate of 40%.

The point estimate is the mean of the two bounds of the confidence interval. So

`P = (5304 + 8686)/(2) = 6955`

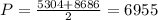
The point estimate is 6995.

b. Calculate the margin of error.

The margin of error is the difference between the bounds and the point estimate. So

M = 8686 - 6995 = 6995 - 5304 = 1691

The margin of error is of 1691.

c. Calculate the standard error of the prediction of student expenditures at a university with a graduation rate of 40%.

Now I have to expand a bit into the confidence interval.

We have that to find our
`\alpha` level, that is the subtraction of 1 by the confidence interval divided by 2. So:

`\alpha = (1 - 0.9)/(2) = 0.05`

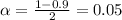
Now, we have to find z in the Ztable as such z has a pvalue of
`1 - \alpha`.

That is z with a pvalue of
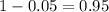`1 - 0.05 = 0.95`, so Z = 1.645.

The margin of error is:

`M = zs`

In which s is the margin of error.

We have that M = 1691. So

`M = zs`

`s = (M)/(z) = (1645)/(1.645) = 1000`

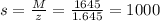
The standard error is of 1000