Answer:
He invested $32000 in the 5% account and $8000 in the 7% account
Explanations:
Total amount invested in both accounts = $40000
Let the amount invested in the 5% account be x
The amount invested in the 7% account = 40000 - x
Total interest = $2160
Let the interest on the 5% account be y
The interest on the 7% account = 2160 - y
time, T = 1 year
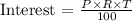
where P is the prinicipal, R is the rate, and T is the time
For the 5% account:
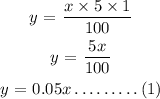
For the 7% account:
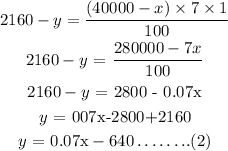
Equate (1) and (2)
0.05x = 0.07x - 640
0.07x - 0.05x = 640
0.02x = 640
x = 640/0.02
x = 32000
Amount invested in the 5% account = $32000
Amount invested in the 7% account = 40000 - x
Amount invested in the 7% account = $40000 - $32000
Amount invested in the 7% account = $8000