Question

Translate and solve the inequality. “Ten subtracted from the quotient of a number and seven is less than negative six.”

Answer 1

Given:

The objective is to translate the statement and solve the inequality.

Step-by-step explanation:

Consider the unknown number as x. The quotient of a number and 7 can be written as,

`Q=(x)/(7)\text{ . . . . .. . (1)}`

By subtracting 10 from the quotient, the value is less than -6.

`Q-10<-6\text{ . . . . .(2)}`

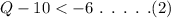
On substituting the equation (2) in (1),

`(x)/(7)-10<6\text{ . . . . . . (3)}`

To find x :

On solving the equation (3), the value of x can be calculated as,

`\begin{gathered} (x-10(7))/(7)<-6 \\ x-70<-6(7) \\ x<-42+70 \\ x<28 \end{gathered}`

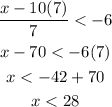
Hence, the value of x is less than 28.