Question

If TR = 11 ft, find the length of PS. Round to the nearest hundredth.PTR16°Sarc PS =ft

Answer 1

Given:

TR = 11 Ft

∠RTS = 16°

Let's determine the length of Arc PS,

Step 1: Let's first determine the angle of Arc PS.

∠RTS = 16°

∠PTQ = 16° ; Vertical angle pair of ∠RTS

Since ∠QTR = ∠PTS under the rule of vertical angles, we can now determine the measure of ∠PTS or Arc PS.

We get,

`\angle RTS\text{ + }\angle PTQ\text{ + }\angle QTR\text{ + }\angle PTS=360`
`16\text{ + }16\text{ + }\angle PTS\text{ + }\angle PTS=360`
`32\text{ + }2\angle PTS=360^{}`
`\angle PTS=\frac{360^{}\text{ - 32}}{2}`
`\angle PTS=(328)/(2)`
`\angle PTS=164^(\circ)`

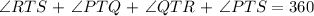
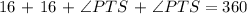
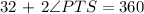
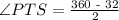
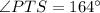
Step 2: Let's determine the perimeter of the circle.

`\text{ Perimeter = }2\pi r`
`\text{ = 2}\pi(11)`
`\text{ Perimeter = 22}\pi`

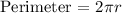
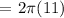
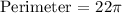
Step 3: Let's determine the length of Arc PS.

`\text{ Arc Length = (}(\theta)/(360))(\text{Perimeter of the Circle)}`
`\text{ = (}(164)/(360))(22)(3.14)`
`\text{ Arc Length = }31.469777\ldots\text{ }\approx\text{ 31.47 ft.}`

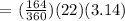
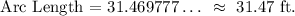
Therefore, the length of Arc PS is 31.47 ft.