Question

Type the correct answer in the box. Use numerals Instead of words. If necessary, use the fraction bar.

Answer 1

We must find the value k such that Q(x) = (x+2) is a factor of the cubic polynomial:

`P(x)=x^3-6x^2-11x+k`

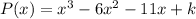
Now, if (x+2) is a factor of the polynomial P(x), then the rest R of the following division must be zero,

`(P(x))/(Q(x))`

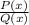
So we must compute the division between the polynomials and check the condition to have R = 0. We compute the quotient by applying the method of synthetic division. Doing that we have:

From the division we see that the rest is:

`R=k-10`

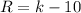
The condition is that the rest R must be equal to zero, so:

`R=k-10=0\Rightarrow k=10`

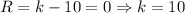
Answer: k = 10