The Equation of a Circle
Given a circle of the center at (h,k) and radius r, the equation of the circle can be written as follows:

The circle has the following properties:
Center at (-8,8). Thus h=-8 and k=8
Radius:
![r=3\sqrt[]{3}](https://img.qammunity.org/2023/formulas/mathematics/college/qy9yho8tv251bvfzrlbiegquosqdnt2kyg.png)
Substituting in the equation above:
![(x+8)^2+(y-8)^2=(3\sqrt[]{3})^2](https://img.qammunity.org/2023/formulas/mathematics/college/si0pgt23tnb0t3pfizhvd0vy32z8y9fh6i.png)
To express this equation in general form, we must expand and operate all the squares:
![x^2+16x+64+y^2-16y+64=3^3(\sqrt[]{3})^2=9\cdot3=27](https://img.qammunity.org/2023/formulas/mathematics/college/3a6jjip8qq020aj3fp4tf4o9qihusip5ql.png)
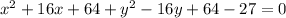
Simplifying:
