Question

Solve the system by elimination 5x -3y + 4z = - 4- 4x + 2y - 3z = 3- x +5y + 7z = 13X =Y =Z =

Answer 1

X=-1

Y=1

Z=1

Step-by-step explanation

`\begin{gathered} 5x-3y+4z=-4\Rightarrow equation(1) \\ -4x+2y-3z=3\Rightarrow equation(2) \\ -x+5y+7z=13\Rightarrow equation(3) \end{gathered}`

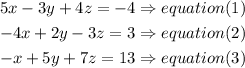
Step 1

in order to eliminate y,

a))Mutiipy equation (3) by 5, then add the new equation to equation (1)

`\begin{gathered} -x+5y+7z=13\text{ }\Rightarrow eq(3)\text{ by 3} \\ 5x-3y+4z=-4\text{ }\Rightarrow eq(3)\text{ by 5} \\ \text{so} \\ -3x+15y+21z=39 \\ 25x-15y+20z=-20 \\ _(-------------------) \\ 22x+41z=19\Rightarrow equation(4) \end{gathered}`

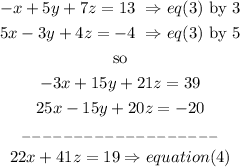
so

now, equation(1) by 2, added to equaiton (2) by 3

`\begin{gathered} 5x-3y+4z=-4\Rightarrow equation(1)\cdot2 \\ -4x+2y-3z=3\Rightarrow equation(2)\cdot3 \\ so \\ 10x-6y+8z=-8 \\ -12x+6y-9z=9 \\ _(-----------------) \\ -2x-z=1\Rightarrow equation\text{ (5)} \end{gathered}`

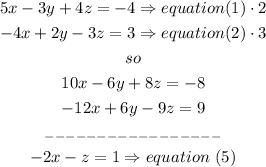
Step 2

now, use equation(4) and equation (5) to find x and z

`\begin{gathered} 22x+41z=19\Rightarrow equation(4) \\ -2x-z=1\Rightarrow equation\text{ (5)} \end{gathered}`

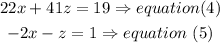
a)in order to eliminate x, multiply equation (5) by 11, then add the result to equation(4)

`\begin{gathered} -2x-z=1\Rightarrow equation\text{ (5) by 11} \\ -22x-11z=11 \\ ad\text{d to equation(4)} \\ -22x-11z=11 \\ 22x+41z=19 \\ ------------ \\ 30z=30 \\ so \\ z=(30)/(30)=1 \\ z=1 \end{gathered}`

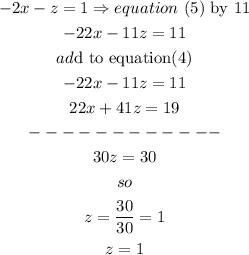
hence

Z= 1

Step 3

now, replace the z value into equation(5) and isolate x

`\begin{gathered} -2x-z=1\Rightarrow equation\text{ (5)} \\ \text{replace} \\ -2x-(1)=1 \\ -2x-1=1 \\ add\text{ 1 in both sides} \\ -2x-1+1=1+1 \\ -2x=2 \\ \text{divide both sides by -2} \\ (-2x)/(-2)=(2)/(-2) \\ x=-1 \end{gathered}`

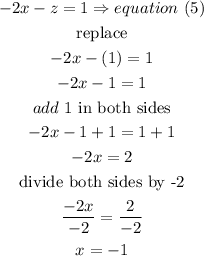
so

X=1

Step 4

finally, replace X and Z value into equation (1), then solve for x

`\begin{gathered} 5x-3y+4z=-4\Rightarrow equation(1) \\ 5(-1)-3y+4(1)=-4 \\ -5-3y+4=-4 \\ \text{add like terms} \\ -3y-1=-4 \\ \text{add 1 in both sides} \\ -3y-1+1=-4+1 \\ -3y=-3 \\ y=(-3)/(-3) \\ y=1 \end{gathered}`

therefore,

Y=1

so, the answer is

X=-1

Y=1

Z=1

I hope this helps you