Question

What is the area of a parallelogram if the coordinates of its vertices are (-4,-1), (-2,4), (4,4) and (2,-1)?

Answer 1

- To solve this problem, the best course of action is to first plot the points of the parallelogram on a coordinate plane.

- Next, we can trace out the dimensions of the parallelogram from the coordinate plane.

- Then, we can find the area of the parallelogram using the formula given below:

`A=\text{base}*\text{height}`

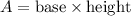
Solution

Plotting the coordinates:

Dimensions of the parallelogram;

The only two dimensions we need here are the base of the parallelogram and the height.

These are illustrated in the diagram below:

Thus, we can conclude that:

height = 5 units

base = 6 units.

Find the area of the parallelogram:

`\begin{gathered} \text{Area}=\text{base}*\text{height} \\ \text{Area}=5*6 \\ \\ \therefore\text{Area}=30\text{units}^2 \end{gathered}`

Answer

The area of the parallelogram is 30 square units