Question

Hello my daughter needs help with numbers 7 and 8

Answer 1

Question 7

`42+0+6`

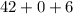
We can find the sum as follow.

We will arrange each value into ones and tens

42= 4 tens + 2 ones

0= 0 ones

6= 6 ones

Hence,

`42+0+6=48`

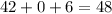
Also, we can use the commutative property of addition,

If we Re-write the expression as

`0+6+42,\text{ we will still get 48 because the order of the addends do not change the sum}`

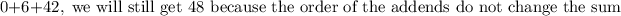
For Question 8

`57+8+3`

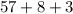
We can re-group as

`\begin{gathered} (57+8)+3=65+3=68 \\ or \\ 57+(8+3)=57+11=68 \end{gathered}`

In both cases, we have 68 to be the answer.

We applied the Associative property of addition: Changing the grouping of the addends does not change the sum.