Question

A restaurant offers the choice of a round pizza with a diameter of 12 inches for $5.65 or a rectangular pizza that measures 12 inches by 24 inches for $11.52. Which is the better buy? (Round the area to the nearest inch and the cost per square inch to the nearest cent.)

Answer 1

Given:

A restaurant offers two choices

For each choice, we will find the unit rate the cost per square inch to the nearest cent

The first choice: a round pizza with a diameter of 12 inches for $5.65

We will find the area of round pizza:

The radius = r = 12/2 = 6 inches

Area = πr² = 3.14 * 6² = 113.04 square inch

So, the unit rate =

`(5.65)/(113.04)\approx0.05`

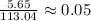
So, the unit rate for the first choice = $0.05 per square inch

The second choice: a rectangular pizza that measures 12 inches by 24 inches for $11.52

The area of the rectangular pizza = 12 x 24 = 288 square inch

so, the unit rate =

`(11.52)/(288)=0.04`

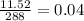
So, the unit rate for the second choice = $0.04 per square inch

So, the better buy will be the lowest cost = $0.04

So, the answer will be the better buy is the second choice:

A rectangular pizza that measures 12 inches by 24 inches for $11.52