Question

What is the slope of the line perpendicular to the line whose equation is x+2y=2 fully simplify

Answer 1

first at all, we need to remember the general equation of the line:

`\begin{gathered} y=mx+b \\ m-\text{slope} \\ b-\text{intercept with y axis} \end{gathered}`

Now, we solve the given equation to y, like this:

`\begin{gathered} x+2y=2 \\ 2y=-x+2 \\ y=-(1)/(2)x+(2)/(2) \\ y=-(1)/(2)x+1 \end{gathered}`

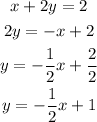
Fron that equation, we conclude that the slope is -1/2

Later, we need to remember that two lines are perpendiculars when the product of its slopes is -1. It means:

`\begin{gathered} m_1\cdot m_2=-1 \\ \text{if m}_1=(-1)/(2),\text{ then:} \\ -(1)/(2)\cdot m_2=-1 \\ m_2=(-1)\cdot(-2) \\ m_2=2 \end{gathered}`

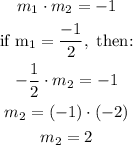
Finally, the slope of the line perpendicular to the line whose equation is x+2y=2 is 2.