Question

A charge of 3.947 nC is moved from a position on the y axis of 8.216 cm to a position on the x axis of 2.848 cm while there is a charge 35.083 nC located at the origin. How much work in micro-Joules did it take to move the charge?

Answer 1

Given:

The charge on the Y-axis is,

`\begin{gathered} q_1=3.947\text{ nC} \\ =3.947*10^(-9)\text{ C} \end{gathered}`

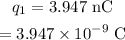
The initial position of the charge is,

`\begin{gathered} y=8.216\text{ cm} \\ =0.08216\text{ m} \end{gathered}`

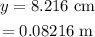
The final position of the charge is,

`\begin{gathered} x=2.848\text{ cm} \\ =0.02848\text{ m} \end{gathered}`

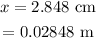
The charge at the origin is,

`\begin{gathered} q_2=35.083\text{ nC} \\ =35.083*10^(-9)\text{ C} \end{gathered}`

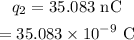
To find:

The work to move the charge

Step-by-step explanation:

The potential at the first point is,

`\begin{gathered} V_1=(kq_2)/(y) \\ k=9*10^9\text{ N.m}^2.C^(-2) \end{gathered}`

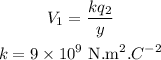
The potential at the final point is,

`V_2=(kq_2)/(x)`

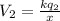
The work in this process is,

`\begin{gathered} W=q_1(V_2-V_1) \\ =kq_1q_2((1)/(x)-(1)/(y)) \\ =9*10^9*3.947*10^(-9)*35.083*10^(-9)((1)/(0.02848)-(1)/(0.08216)) \\ =2.86*10^(-5) \\ =28.6*10^(-6)\text{ J} \\ =28.6\text{ }\mu J \end{gathered}`

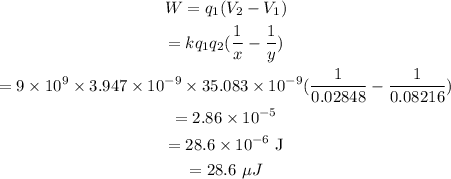
Hence, the work is,

`28.6\text{ }\mu J`