Given the system of equations :
8x + 16y = 15 →(1)
x = 5 - 2y →(2)
By substitution with x from equation (2) at the equation (1) :
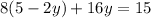
Solve for y :
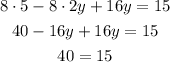
The last result is un logic , which mean the given system of equation represents two lines with the same slope and different y- intercepts
So, the given system represents a parallel lines
So, the answer is option C. the solution set is the empty set