Question

I was helped with a similar question earlier this week for my study guide. But I'm confused on how to place the numbers on the number line

Answer 1

Given the data:

5.6, 5.5, 5.1, 5.3, 5.2, 5.5, 5.6, 5.2, 5.7, 5.2

Let's draw a box plot using the given diameters.

A box plot has 5 number summary, which are:

Minimum, first quartile (Q1), median, thrid quartile(Q3), maximum.

It is represented below:

Hence, we have:

• Minimum: ,This is the smallest data value.

Therefore, the minimum is 5.1

• First Quartile (Q1):

The Q1 is the median of the lower half of the data.

Arrange the number s in ascending order:

5.1, 5.2, 5.2, 5.2, 5.3, 5.5, 5.5, 5.6, 5.6, 5.7

Lower half of data:

5.1, 5.2, 5.2, 5.2, 5.3

The median of the lower half is = 5.2

Therefore, the Q1 = 5.2

• Median:

Median is the middle data.

Arrange the number s in ascending order:

5.1, 5.2, 5.2, 5.2, 5.3, 5.5, 5.5, 5.6, 5.6, 5.7

The two middle numbers are:

5.3 and 5.5

SInce we have two middle numbers, the median will be the average of the two middle numbers.

`(5.3+5.5)/(2)=(10.8)/(2)=5.4`

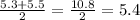
Therefore, the median is 5.4

• Third Quartile:

The Q3 is the median of the upper half of the data.

Arrange the number s in ascending order:

5.1, 5.2, 5.2, 5.2, 5.3, 5.5, 5.5, 5.6, 5.6, 5.7

Upper half of data:

5.5, 5.5, 5.6, 5.6, 5.7

Median of upper half = 5.6

Therefore, the third quartile, Q3 = 5.6

• Maximum:

The maximum is the greatest number in the data set.

The maximum is 5.7

Therefore, the five number summary is:

• Min = 5.1

,

• Q1 = 5.2

,

• Median = 5.4

,

• Q3 = 5.6

,

• Max = 5.7

Therefore, the box plot is below: