Question

Please help me find CE. I am stressing over this.

Answer 1

We are asked to find the arc CE in the given figure.

First, we need to find arc mBC then we can easily find arc CE

As you can see from the figure, ∠BAC is formed by two secants intersecting outside of circle.

The intercepted arcs are mDE (major arc) and mBC (minor arc)

So, we can use the property of "angle Formed by Two Secants" given by

`\angle BAC=(1)/(2)(m\bar{DE}-m\bar{BC)}`

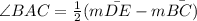
Where

∠BAC = 20°

mDE = 100°

Let us solve the above equation for mBC

`\begin{gathered} \angle BAC=(1)/(2)(m\bar{DE}-m\bar{BC)} \\ 20\degree=(1)/(2)(100\degree-m\bar{BC)} \\ 2\cdot20\degree=100\degree-m\bar{BC} \\ 40\degree=100\degree-m\bar{BC} \\ m\bar{BC}=100\degree-40\degree \\ m\bar{BC}=60\degree \end{gathered}`

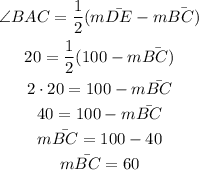
Now we can find the arc CE

`\begin{gathered} m\bar{BC}+m\bar{BD}+m\bar{DE}+\bar{mCE}=360\degree \\ 60\degree+100\degree+100\degree+\bar{mCE}=360\degree \\ 260\degree+\bar{mCE}=360\degree \\ \bar{mCE}=360\degree-260\degree \\ \bar{mCE}=100\degree \end{gathered}`

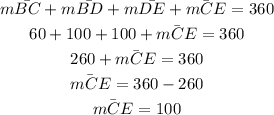
Therefore, the arc CE is 100°