Question

Given the binomial experiment with n = 100 trials and probability of success on a single trial p = 0.09, find the value of = Use the Poisson distribution to estimate the probability of r = 9 successes. (Round your answer to four decimal places.)

Answer 1

The value of = 9

The probability of r=9 successes = 0.1318

Step - by - Step Explanation

What to find?

• The value of .

,

• The probability of r=9 successes.

Given parameters:

• The number of trials, n = 100

,

• Probability of success on a single trial, P=0.09

,

• probability of r = 9

To find the value of , we will use the formula below:

= np

susbtitute the values and substitute.

= 100 x 0.09

= 9

The probability of r=8 successes is given by P(r = 8).

The formula for computing the poisson distribution is given by;

`P(r)=(e^(-\lambda)\lambda^r)/(r!);\text{ r=}0,\text{ 1, 2 }\ldots`

Substitute the values and simplify.

`P(9)=(e^(-9)(9)^9)/(9!)`

`P(9)=(47811.4850634)/(362880)`

`=0.13175564001`

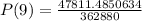
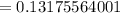
Hence, the probability of r=9 successes is 0.1318