ANSWER
Yes, the sample mean is more than the population mean
Step-by-step explanation
We need to find out if the sample mean is more than the population mean.
The population mean is the mean of the entire data of pets in the neighborhood.
The sample mean is the mean of the sample of data.
The mean of a set of data is the sum of the entire data divided by the total number of data points.
The population mean is therefore:
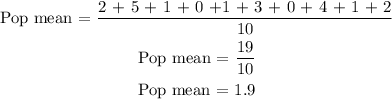
The sample mean is therefore:
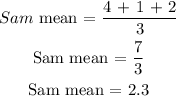
Therefore, the sample mean is more than the population mean.