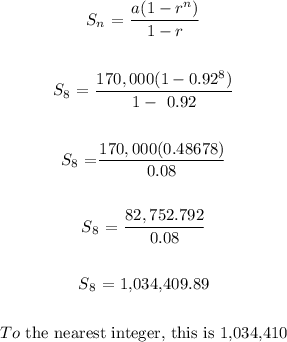
The number of Fishes caught by the company over the first 8 years is 1,034,410 fishes
Here, we want to find the sum of the fishes the company was able to catch over the first 8 years
We start by setting up an exponential equation to represent the number of fishes caught at any number of years in time
We can have the exponential equation as;
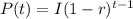
where I is the population of fishes in the first year
r is the percentage decrease which is 8% and it is same as 8/100 = 0.08
t is the year number
and P(t) is the population at a certain year
So substituting these values, we have;
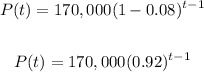
So in this case, we have a geometric series with the nth term given above such that;
The first term a, is 170,000
The common ratio is 0.92
So the sum of the first 8 years which is the sum of the first 8 terms can be obtained using the formula for the geometric series as follows;