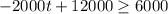Question

Suppose a business purchases equipment for $12,000 and depreciates it over 5 years with the straight-line method until it reaches its salvage value of $2000 (see the figure below). Assuming that the depreciation can be for any part of a year, answer the questions to the right.

Answer 1

We have the next points

(0,12000)=(x1,y1)

(2,8000)=(x2,y2)

First, we need to find the slope

`m=(y_2-y_1)/(x_2-x_1)`

we substitute the values

`m=(8000-12000)/(2-0)=(-4000)/(2)=-2000`

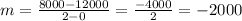
then we know that the y-intercept is 12000

where V represents the y-axis and t represents the x-axis

The equation that represents the depreciated value V as a function of t is

V=-2000t+12000

Then for the inequality, that depreciated value of V is less than 6000

`-2000t+12000<6000`

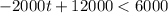
Then for the inequality that describes the time t during which the depreciated values is at least half of the original, half value of the original is 12000/2=6000

t= 3 when V= 6000

therefore

`-2000t+12000\ge6000`