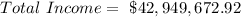Question

Suppose you go to a company that pays $0.04 for the first day, $0.08 for the second day, $0.16 for the third day, and so on.If the daily wage keeps doubling, what will your total income be for working 30 days?Total Income = $

Answer 1

Given that the company pays $0.04 for the first day, $0.08 for the second day, $0.16 for the third day, and so on, you can identify this Geometric Sequence:

`0.04,0.08,0.16...`

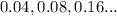
Where the first term is:

`a=0.04`

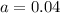
And the Common Ratio is:

`r=2`

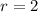
Because the daily wage keeps doubling.

In order to calculate your total income for working 30 days, you can use the following formula for the Partial Sum of the first "n" terms of the Geometric Sequence:

`S_n=(a(1-r^n))/(1-r)`

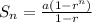
Where "a" is the first term and "r" is the Common Ratio. Remember that:

`r\\e1`

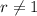
In this case:

`n=30`

Therefore, when you substitute values into the formula and evaluate, you get:

`S_(30)=42,949,672.92`

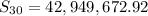
Hence, the answer is:

`Total\text{ }Income=\text{ \$}42,949,672.92`