Okay, here we have this:
Considering the provided information we obtain the following when we replace the point in the function:
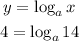
Now, let's solve for a:
![\begin{gathered} 4=(1)/(\log_(14)\left(a\right)) \\ 4\log _(14)\mleft(a\mright)=1 \\ \log _(14)\mleft(a\mright)=(1)/(4) \\ a=\sqrt[4]{14} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/dsayj58oivyuquvszi34fzu59ibcq3dtxx.png)
Finally we obtain that a is equal to ⁴√14. We can check it in the following graph resulting from the function: