Question

A tree stands betweenAlice andBob, who are 200 feet apart. Alice reports the angle of elevationof the trees is 27º and Bob reports that it is 18º. How far is Alice from the tree?

Answer 1

A little schematic will help us in understanding the situation.

Now, we are looking for the distance, h, between Alice and F

First, we need to find our distance, AT.

AT is a side in the triangle ATB and can be found with the sine rule since we have at least 2 angles.

`\begin{gathered} (AB)/(\sin T)=(AT)/(\sin B) \\ AT=(AB\sin B)/(\sin T) \\ AT=(200\sin18)/(\sin135)=87.4^(\prime) \end{gathered}`

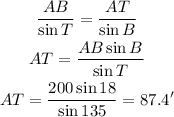
AT is a side in the right-angled triangle AFT.

We will use the SOH CAH TOA to get our value of h.

`\begin{gathered} \cos A=(h)/(AT) \\ h=AT\cos A \\ h=87.4\cos 27=77.877^(\prime) \end{gathered}`

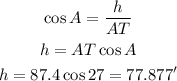
Alice is 77.88' away from the tree