The period of a tangent function y = a tan(bx) is given by the distance between consecutive x-intercepts.
From the given graph, 2π and 7π/4 are consecutive x-intercepts.
Therefore, the period T of the function is given by:

The frequency f given the period T is :
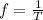
Since T = π/4, it follows that:
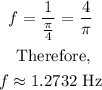
Therefore, the required frequency is approximately 1.2732 Hz