We have to simplify the expression:

The second parenthesis has a "-" sign at the front. So, all the signs of each term within the parenthesis changes.
We have:
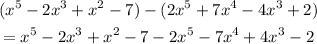
Now, we can simplify the expression by adding/subtracting like terms (terms whose variables and exponents are the same). This process of simplification is shown below:
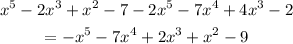
Answer
