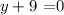Question

Find the equation containing the given point. Write the equation in slope-intercept form (7,-9)(9,-9)

Answer 1

Step 1:

In this question, we are given the following:

Find the equation containing the given point.

Write the equation in slope-intercept form:

`\left(7,\text{ -9 \rparen and \lparen 9, -9 \rparen}\right?`

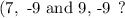
Now, we are to find the gradient of the two points:

`Gradient\text{ , m =}(y_2-y_1)/(x_2-x_1)`
`Gradient,\text{ m =}(-9-\left(-9\right))/(9-7)=(-9+9)/(2)=(0)/(2)=0`

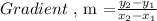
Using the equation,

`y-y_1=\text{ m \lparen x-x}_1)`
`y\text{ - \lparen-9\rparen = 0 \lparen x -\lparen-9\rparen \rparen}`
`y+9=0`

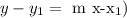
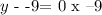
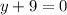
The graph showing the slope-intercept form,

CONCLUSION:

The equation in slope-intercept form =

`y+9\text{ =0}`